
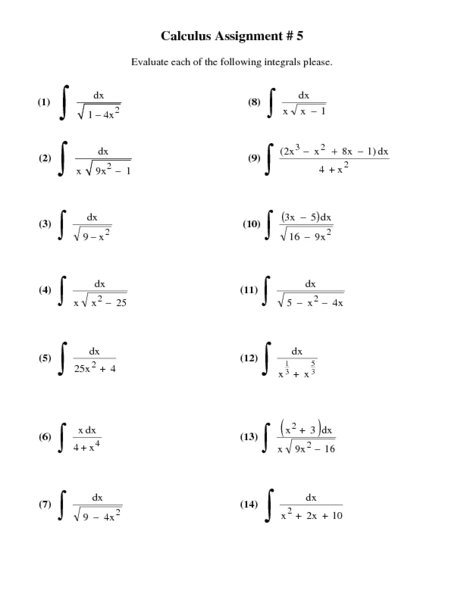
We can then finally use a no-arbitrage argument to price a European call option via the derived Black-Scholes equation. In order to price our contingent claim, we will note that the price of the claim depends upon the asset price and that by clever construction of a portfolio of claims and assets, we will eliminate the stochastic components by cancellation. We start at the very beginning with a refresher on the rise over run formulation of a slope, before converting this to the formal definition of the gradient of a function. We will form a stochastic differential equation for this asset price movement and solve it to provide the path of the stock price. This course offers a brief introduction to the multivariate calculus required to build many common machine learning techniques. A geometric Brownian motion is used instead, where the logarithm of the stock price has stochastic behaviour. A standard Brownian motion cannot be used as a model here, since there is a non-zero probability of the price becoming negative. To better understand and have an idea regarding. To solve the calculus, first, know the concepts of limits.
Each function has a relationship among two numbers that define the real-world relation with those numbers.
A vanilla equity, such as a stock, always has this property. These key points are: To understand the basic calculus formulas, you need to understand that it is the study of changing things. For this we need to assume that our asset price will never be negative. In the subsequent articles, we will utilise the theory of stochastic calculus to derive the Black-Scholes formula for a contingent claim. This operation has a name: it is called the square. This is an operation that acting on 25 25 should give back 5 5. We can ask for the inverse of this squaring operation. This is the operation of multiplying a number by itself. The derivative of a random variable has both a deterministic component and a random component, which is normally distributed. Among the operations of multiplication is that of squaring a number. All it ever does is taking a line of letters (or symbols), and performing a little cut and paste operation on it. This concerns rates of changes of quantities and slopes of curves or surfaces in 2D or multidimensional space. It can be broadly divided into two branches: Differential Calculus. Don’t be intimidated by the word calculus It does not have any complicated formulae or operations. Calculus is a study of rates of change of functions and accumulation of infinitesimally small quantities. The fundamental difference between stochastic calculus and ordinary calculus is that stochastic calculus allows the derivative to have a random component determined by a Brownian motion. The Lambda Calculus has been invented at roughly the same time as the Turing Machine (mid-1930ies), by Alonzo Church. Ito's Lemma is a stochastic analogue of the chain rule of ordinary calculus. A fundamental tool of stochastic calculus, known as Ito's Lemma allows us to derive it in an alternative manner. The Binomial Model provides one means of deriving the Black-Scholes equation.
This process is represented by a stochastic differential equation, which despite its name is in fact an integral equation. Let us Find a Derivative To find the derivative of a function y f(x) we use the slope formula. The physical process of Brownian motion (in particular, a geometric Brownian motion) is used as a model of asset prices, via the Weiner Process. The main use of stochastic calculus in finance is through modeling the random motion of an asset price in the Black-Scholes model. In quantitative finance, the theory is known as Ito Calculus. Properties of the various kinds of numbers we will encounter are reviewed. Instead, a theory of integration is required where integral equations do not need the direct definition of derivative terms. This rules out differential equations that require the use of derivative terms, since they are unable to be defined on non-smooth functions. Many stochastic processes are based on functions which are continuous, but nowhere differentiable. We hope that you find these tutorials useful.Stochastic calculus is the area of mathematics that deals with processes containing a stochastic component and thus allows the modeling of random systems.
Basics of calculus for beginners series#
This series is a thorough approch to mathematics that begins with basic arithmatic and ends with vector calculus. If a function f is differentiable in the interval of consideration, then f’ is defined in that interval. It is mostly useful for the following two purposes: To calculate f from f’ (i.e. All of the YouTube videos on this page were created and posted by Professor Dave Explains. Integral calculus is the study of integrals and their properties.


 0 kommentar(er)
0 kommentar(er)
